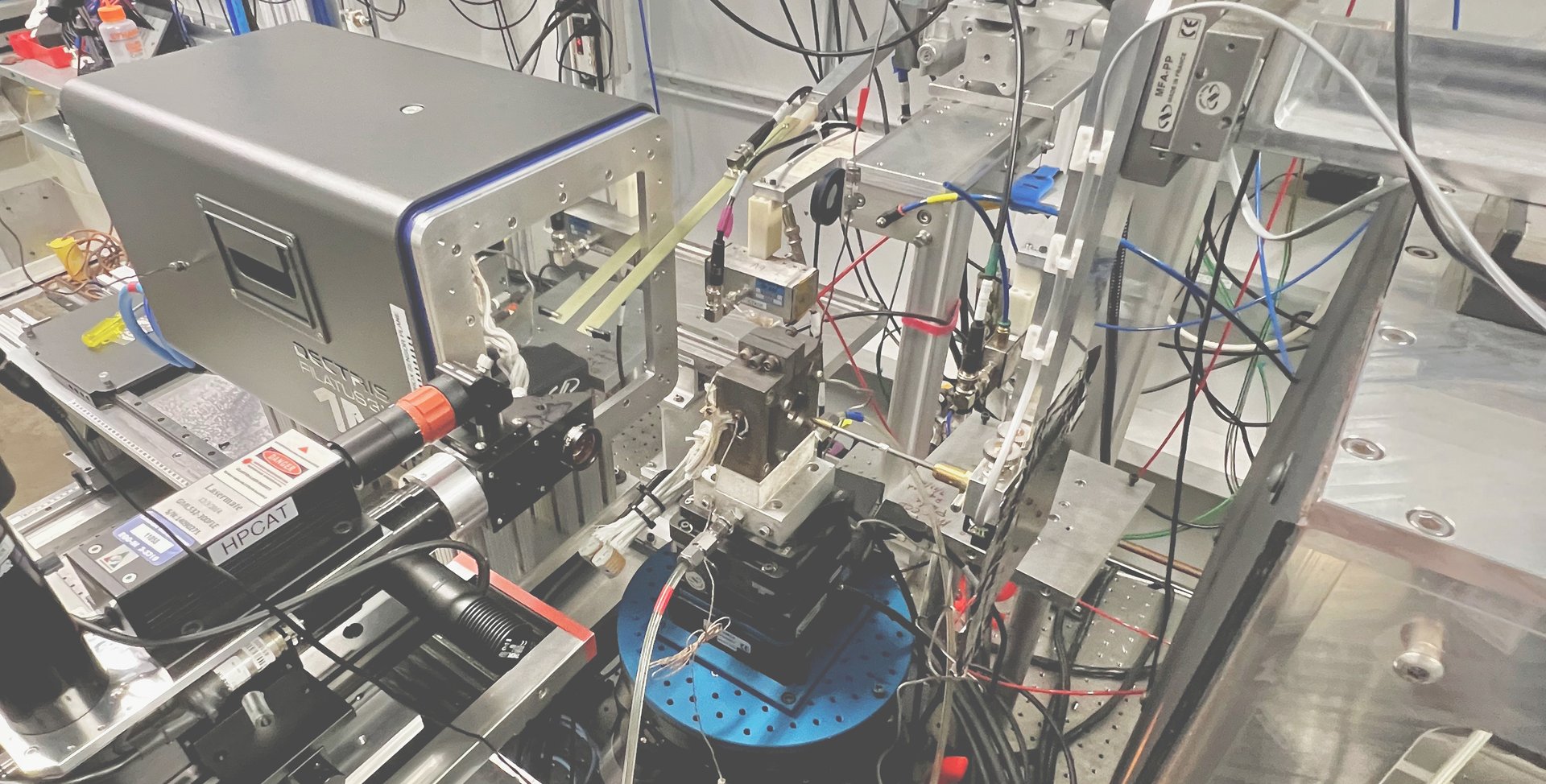
science | Stefan Lohaus, scientist, research, Caltech, ETH Zurich, Physics, Chemistry

X-ray diffraction experiment at the Advanced Photon Source, an ultra-bright synchrotron at Argonne National Laboratory, IL. X-rays come out of the needle-like aperture, go through the sample inside a heating block, and scatter into the area detector on the left.

Molecular quantum information science

Imagine a sensor so small that it fits into individual molecules and binds to proteins or DNA to diagnose diseases at the atomic scale. What if nature already has such sensors, and all we need to do is learn how to use them?

When electrons spin, they create localized magnetic fields that are sensitive to their environment. In the quantum realm, the direction of the electronic spin (and consequently the magnetic field) is quantized, restricted to two discrete orientations: "up" or "down." This binary nature mirrors the fundamental units of classical computing, the "0" and "1" bits of information. However, quantum mechanics introduces a mind-boggling twist: an electron's spin can exist in both states at the same time. Yes, electrons exhibit two seemingly contradictory behaviors simultaneously, a phenomenon known as quantum superposition. This property makes a quantum bit of information, or qubit, vastly more powerful than a classical binary bit. Multiple computations can be performed in parallel by processing all possible quantum superpositions at once.

Quantum information science extends beyond miniaturized computing; it enables applications in quantum communication and biological sensing since superpositions are delicate and highly affected by their surroundings. When the qubit is a molecule, chemists know how to attach them to various biological and physical systems. However, a critical challenge in realizing practical quantum technologies is extending the lifespan of quantum superposition states, which is restricted to only a few microseconds in molecules. Cooling molecules to near absolute zero slows down decoherence processes, but who wants to be frozen for a medical diagnosis? At the atomic scale, thermal energy disrupts quantum states—atoms vibrate, electrons transition between energy levels, and spins lose coherence, erasing quantum information.

This is where my expertise comes into play. I specialize in measuring atomic vibrations, known as phonons in quantum mechanics. At room temperature, these phonons are the primary factor causing electronic spins to lose coherence. To study these vibrational motions, I employ inelastic neutron scattering at the Spallation Neutron Source of Oak Ridge National Laboratory, nestled near the Smoky Mountains of Tennessee. Neutrons (massive, uncharged particles) are accelerated at a sample, where they transfer energy and momentum into atomic vibrations.

Through these experiments, I seek to understand precisely how molecular vibrations interact with electronic spins to identify if specific vibrational modes significantly influence spin coherence. The ultimate goal is to establish design principles that enable chemists to synthesize molecules with prolonged quantum superpositions for longer sensing and processing times. Traditionally, the study of atomic vibrations and neutron scattering has been confined to physics and materials science. Bridging from these disciplines into chemistry has allowed me to approach molecular systems with a fresh perspective, which has been both scientifically productive and deeply rewarding.

RESEARCH

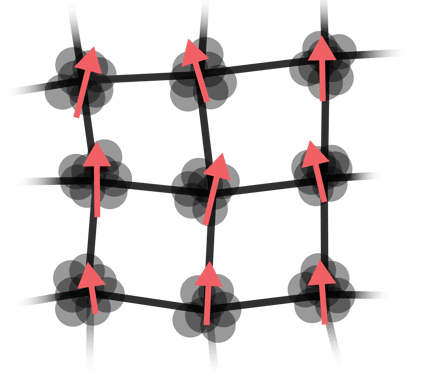
Magnetic fields are created by the spin of electrons in a molecule. Being a quantum system, the direction of the field can be "up", "down", or any combination (superposition) of both directions simultaneously. This superposition of spin states can be created and manipulated in the lab by pulses of microwaves.

Since they are highly affected by their environment, spin superpositions could be implemented as nanoscale sensors in biological systems.

Thermodynamic behavior of materials

Materials science explores the connections between microscopic excitations of atoms and the macroscopic behavior of materials. Such a bottom-up approach enables a deep understanding of physical phenomena and ideally results in useful technology. Thermodynamics refers to different manifestations of energy in materials. At the atomic scale, thermal energy is absorbed as vibrational motions of atoms, as excitations of electrons, and as disruption of magnetic order. Understanding exactly where energy flows gives us insights into the origins of thermophysical properties of materials.

For example, most materials expand when heated because most of the heat goes into atomic vibrations. Usually, the stronger the atoms oscillate, the more space they occupy, causing a volumetric expansion. However, not all materials behave like this. The anomalous absence of thermal expansion in Invar materials arises from interactions between those atomic vibrations and the heat absorbed by electronic spins. The magnetic fields created by the spinning electrons lose their directionality when heated, and the material loses magnetization. This allows for a volume contraction that precisely cancels the expansion from the vibrational motion. By combining different spectroscopic techniques at a synchrotron at Argonne National Laboratory, I measured these two opposing effects, vibrational and magnetic, for the first time.

Atomic vibrations displace atoms from their equilibrium positions and cause distortions in the lattice structure of materials. The spinning electrons create local magnetic fields (arrows), and when these fields are aligned, the material becomes macroscopically magnetized. Lattice vibrations and electronic spins are not independent, and their coupling can cause interesting anomalous behavior, such as materials without thermal expansion — called Invar materials.

Learn more this project

Molecular quantum information science

When electrons spin in molecules, they create local magnetic fields (up and down arrows). The direction of these fields – and the quantum superposition of those directions – can be used to store, sense, and transmit quantum information. In the lab, these magnetic fields are manipulated using microwave pulses.

Quantum information science explores how atomic-scale (quantum) phenomena can be harnessed to sense, store, and transmit information in fundamentally new ways. The goal is to develop sensors and computing capabilities that classical systems cannot achieve.

I study how the magnetic fields created by spinning electrons in molecules can be used to sense their local microscopic environment. However, the thermal motion of the molecules disturbs these magnetic fields, obstructing their sensing capabilities. I design experiments to understand the interactions between molecular vibrations and the magnetic fields, to enable their use for sensing and storing quantum information.

RESEARCH

Thermodynamic behavior of materials

Understanding the atomic-scale behavior of materials helps explain their macroscopic properties. I studied the various ways energy is microscopically absorbed and stored in materials by atomic vibrations, electron excitations, and local magnetic fields. Properties like heat capacity, thermal expansion, and elastic moduli are large-scale manifestations of these microscopic dynamics.

I investigated the anomalous behavior of materials that do not expand when heated, known as the invar effect. With various experiments at synchrotron facilities, I measured for the first time how the thermal expansion caused by atomic vibrations is precisely counteracted by thermal contraction due to magnetic effects.

6 min read

Why do some metals not expand when heated?

My experimental investigation of a long-standing open question in materials science.

Microscopically, thermal energy is absorbed mostly as atomic vibrations, distorting the periodic structure of materials. In magnetic materials, part of the energy also disturbs the alignment of local magnetic fields (red arrows), causing the loss of macroscopic magnetization. Interactions of the magnetic structure with atomic vibrations can give rise to anomalous behaviors, like the invar effect.

Disordered (amorphous) materials

Some materials can lose their periodic crystal structure and become disordered at the atomic scale. This affects a range of macroscopic properties, from hardness and viscosity to thermal transport. Silica glasses are quintessential examples of such amorphous materials, but this phenomenon is also seen in polymers and even in metals that are cooled rapidly enough to prevent crystallization, called metallic glasses.

Stability of large space structures

Imagine collecting solar energy in space and wirelessly transmitting it to Earth, uninterrupted by nighttime or weather conditions. Renewable solar power could become available anywhere, anytime. This is the goal of the Space Solar Power Project, a multidisciplinary collaboration at Caltech. The concept involves assembling arrays of solar collectors on large, flat structures that can be folded origami-style to fit into a rocket. Once in orbit, these ultralight 2D structures unfold, exposing the solar collectors. The collected thermal energy is then converted into microwaves and beamed down to Earth to various receiving stations.

I love the hands-on, experimental side of research. Experiments are at the core of the scientific method. Different experimental techniques allow us to observe different natural phenomena and together with theories, experiments enable the development of models that describe how nature works.

Physicists view phenomena in nature as different manifestations of energy at the atomic scale, and experimental physicists get to measure those. Since we can’t directly see atoms, we use indirect experimental methods. Spectroscopy works by exciting a material with radiation at different energies (think lasers, X-rays, neutrons…), and measuring how the energy of the radiation is altered by the sample, or how it gets diffracted (‘bent’) in different directions after interacting with the sample. All we know about atomic-scale effects is based on this kind of experiment.

EXPERIMENTAL METHODS

These

Inelastic X-ray and neutron scattering

X-ray and neutron diffraction

Mössbauer spectroscopy

Electron paramagnetic resosnance

Calorimetry

Laser Doppler vibrometry

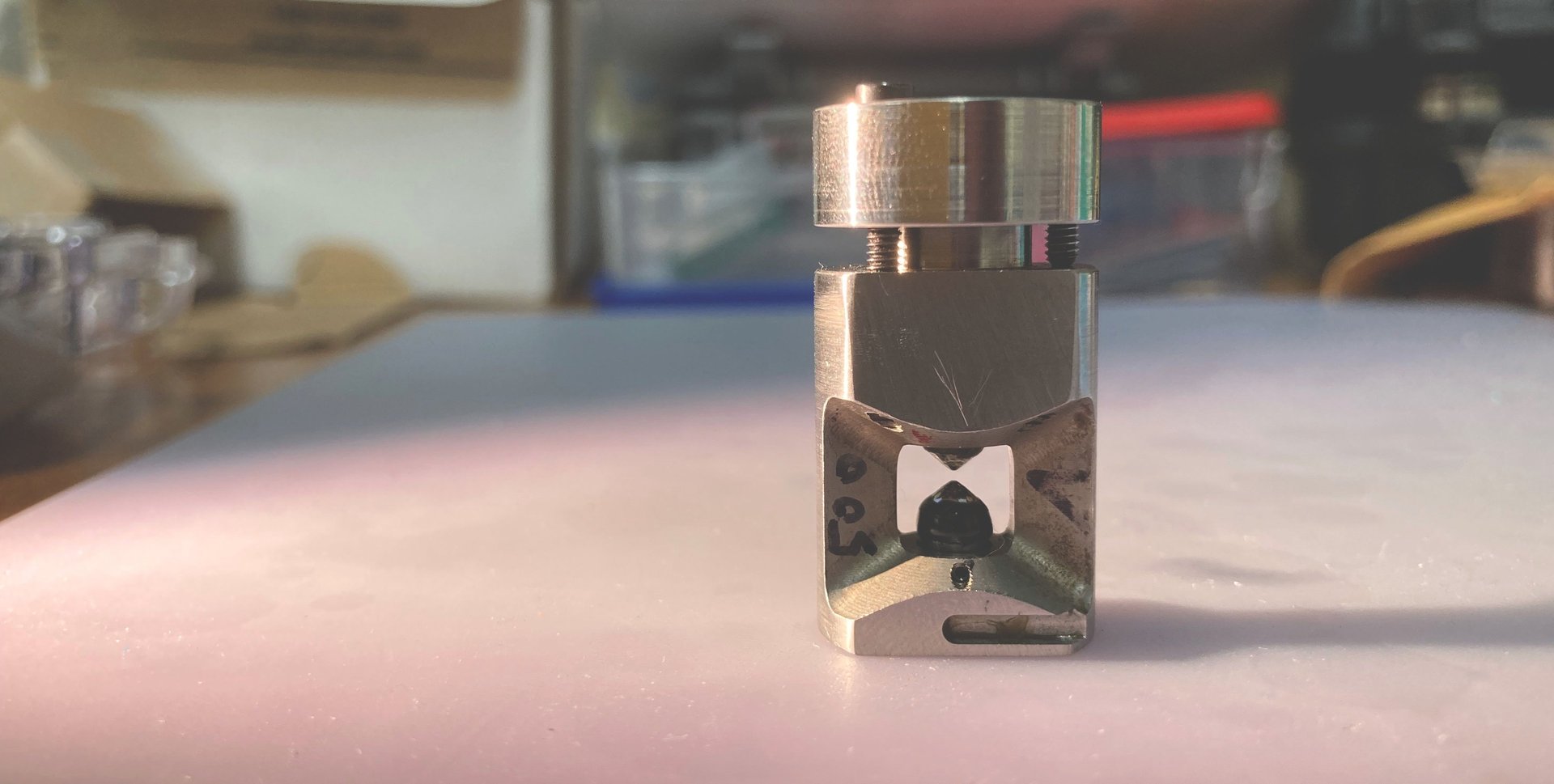
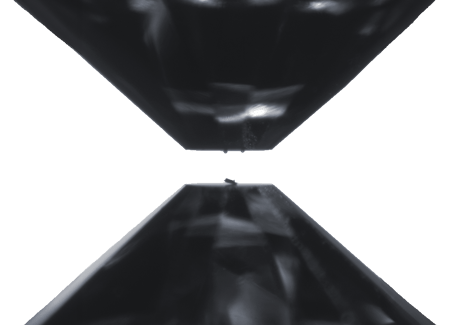
High pressures can be achieved in the lab by using diamond-anvil cells. Two opposing diamonds are pressed against each other, with a sample placed in between them. Being the hardest known natural material, diamonds can withstand pressures found deep inside planetary cores.

147 citations (Google Scholar)

[13] C. N. Saunders, V. V. Ladygin, D. S. Kim, C. M. Bernal-Choban, S. H. Lohaus, G. E. Granroth, D. L. Abernathy, B. Fultz. Diffuse intensity from phonon noise in inelastic neutron scattering. Physical Review Materials 9, 024602 (2025).

[12] C. M. Bernal-Choban, V. Ladygin, G. E. Granroth, C. N. Saunders, S. H. Lohaus, D. L. Abernathy, J. YY. Lin, B. Fultz. Atomistic origin of the entropy of melting from inelastic neutron scattering and machine learned molecular dynamics. Communications Materials 5, 271 (2024).

[11] P. Guzman, S. H. Lohaus, C. M. Bernal-Choban, B. Fultz, J. Y. Zhao, G. Shen, M. Y. Hu, E. E. Alp, B. Lavina. Pressure induced Invar effect in Fe55Ni45: An experimental study with nuclear resonant scattering. Physical Review B 110, 144419 (2024).

[10] S. H. Lohaus, N. P. Kazmierczak, K. M. Luedecke, B. Marx, V. N. Nemykin, R. G. Hadt. Quantifying the effects of peripheral substituents on the spin-lattice relaxation of a vanadyl molecular quantum bit. Journal of Porphyrins and Phthalocyanines 28, 383 (2024) - invited.

[9] S. H. Lohaus, M. Heine, P. Guzman, C. M. Bernal-Choban, C. N. Saunders, G. Shen, O. Hellman, D. Broido, B. Fultz. A thermodynamic explanation of the Invar effect. Nature Physics 19, 1642 (2023) - here is an open-access version.

[8] P. Guzman, C. M. Quine, S. H. Lohaus, R. Toda, V. J. Scott, L. Schull, B. Fultz. A miniaturized piezoelectric Mössbauer spectrometer with feedback control. Review of Scientific Instruments 94, 11 (2023).

[7] S. H. Lohaus. Experimental Study on the Thermodynamic Interactions of Phonons and Magnetism in Fe Systems. PhD Thesis, Caltech Library (2023).

[6] H. L. Smith, C. N. Saunders, C. M. Bernal-Choban, S. H. Lohaus, C. J. Stoddard, L. K. Decker, J.Y.Y. Lin, J. L. Niedziela, D.L. Abernathy, J.-H. Na, M. D. Demetriou, B. Fultz. Vibrational dynamics in the undercooled liquid of ultra-fragile metallic glasses. Materialia 27, 101710 (2023).

[5] S. H. Lohaus*, L. Mauger*, and B. Fultz. The temperature dependence of nuclear resonant x-ray spectra of magnetic iron and cementite. Hyperfine Interactions 243, 1 (2022).

[4] C. N. Saunders, D. S. Kim, O. Hellman, H. L. Smith, N. J. Weadock, S. T. Omelchenko, G. E. Granroth, C. M. Bernal-Choban, S. H. Lohaus, D. L. Abernathy, B. Fultz. Thermal expansion and phonon anharmonicity of cuprite studied by inelastic neutron scattering and ab initio calculations. Physical Review B 105, 174308 (2022).

[3] S. H. Lohaus, M. B. Johnson, P. F. Ahnn, C. N. Saunders, H. L. Smith, M. A. White, B. Fultz. Thermodynamic stability and contributions to the Gibbs free energy of nanocrystalline Ni3Fe. Physical Review Materials 4, 086002 (2020).

[2] M. Delapierre, S. H. Lohaus, S. Pellegrino. Nonlinear vibration of transversely-loaded spinning membranes. Journal of Sound and Vibration 427, 41 (2018).

[1] J. Alonso-Mora, S. H. Lohaus, P. Leemann, R. Siegwart, P. Beardsley. Gesture based human-multi-robot swarm interaction and its application to an interactive display. Proceedings of IEEE International Conference on Robotics and Automation (2015).

PUBLICATIONS

ORCID: 0000-0002-4430-3834

147 citations (Google Scholar)

[7] S. H. Lohaus. Experimental Study on the Thermodynamic Interactions of Phonons and Magnetism in Fe Systems. PhD Thesis, Caltech Library (2023).

[5] S. H. Lohaus*, L. Mauger*, and B. Fultz. The temperature dependence of nuclear resonant x-ray spectra of magnetic iron and cementite. Hyperfine Interactions 243, 1 (2022).

[2] M. Delapierre, S. H. Lohaus, S. Pellegrino. Nonlinear vibration of transversely-loaded spinning membranes. Journal of Sound and Vibration 427, 41 (2018).

PUBLICATIONS

FEATURES

NEWS

SELECTED

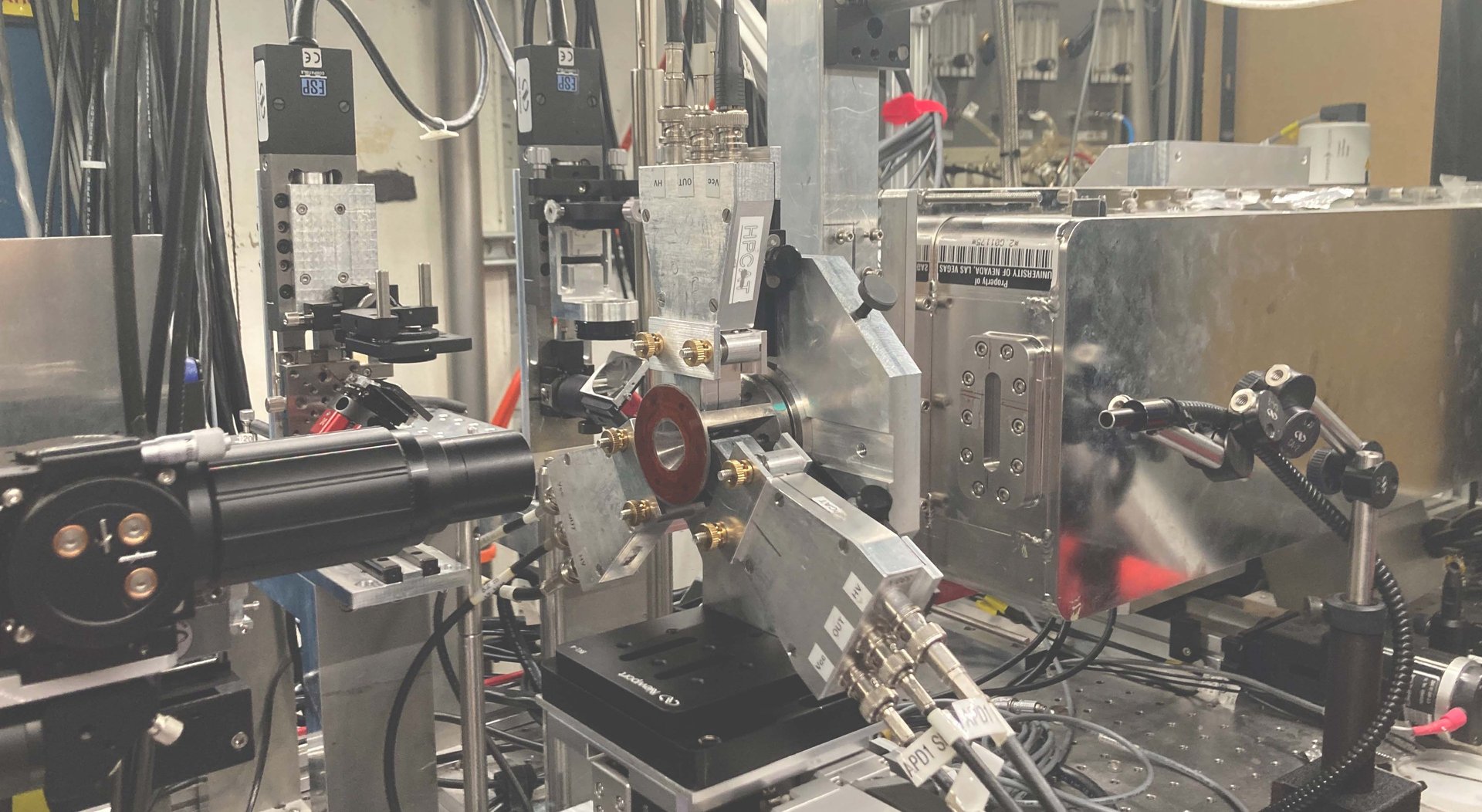
Experimental setup to measure the vibrational motion of atoms and the local magnetization of Invar materials at the Advanced Photon Source, a synchrotron at Argonne National Laboratory - IL. X-rays come out of the beryllium window (metallic box on the right), go through the sample inside the diamond-anvil cell (DAC), and scatter into three detectors placed closely around the DAC.

Why do some metals not expand when heated?

Crystal structure with overlaid snapshots of atomic positions representing the vibrational motion around their equilibrium positions. Heat (thermal energy) induces those vibrations, which cause distortions of the structure. Larger temperatures (right figure) cause larger thermal vibrations and usually result in a volumetric expansion of the lattice.

In 1895, Swiss physicist Charles Édouard Guillaume discovered that combining two cheap and abundant metals, iron and nickel, resulted in an alloy that does not expand when heated. The volume invariance of this material with temperature earned the name invar effect. While this might not seem like the most thrilling scientific discovery, such properties are invaluable in engineered devices that require structural stability against temperature changes. Have you seen photos of train tracks buckling in the heat because of their expansion? Invar alloys are used in everything from large pipelines and tanks to precision instruments such as microscopes, telescopes, and orbiting satellites. Temperature variations also undermine the precision of timekeeping, since the properties of springs inside watches are affected by heat. The discovery of the invar effect revolutionized the watch industry.

Guillaume himself cleverly publicized the invar effect to both Parisians and the academic world using none other than the newly constructed Eiffel Tower. He hung a long invar wire from the second platform down to the ground, 135 meters below, to measure the tower's expansion as temperatures fluctuated over several days. Since the invar wire remained unchanged, it served as a precise length reference to detect small variations of about 2 centimeters. The technical significance of invar, and perhaps Guillaume’s sharp publicity skills, culminated in his 1920 Nobel Prize in Physics. Because of Guillaume, Albert Einstein had to wait another year for his own Nobel, which was awarded in 1921.

Minimizing thermal expansion is technically useful, but the invar effect also raises a puzzling scientific question: How does an alloy of two metals, both of which have substantial thermal expansion, result in a material that does not expand at all? This mystery led to thousands of scientific studies, shedding light on electronic and magnetic aspects that gave pieces of the answer. Guillaume himself suspected that magnetism played a role, as the effect vanished when the metal was heated above its magnetization regime.

Most materials expand when heated. Think of a hot air balloon, or the next time you drive over a bridge, notice the small gaps at both ends designed to accommodate the expansion. But why do materials expand? Simply put, higher temperatures cause atoms to vibrate more intensely, typically requiring more space. These atomic vibrations, known as phonons in quantum mechanics, account for most of a material’s thermodynamic behavior.

This leads to the question: Can this magnetic contraction counteract the thermal expansion caused by atomic vibrations, effectively canceling out expansion altogether? Can these two contributions, magnetic and vibrational, be measured independently to resolve this long-standing question? Or is there another missing piece to the puzzle?

As an experimental scientist, this sounds like an exciting challenge. A fundamental open question in materials science that required experimental proof was right up my alley. But I also understood why it hadn’t been done before. The volume expansion is a bulk property, and individual atomic-scale contributions cannot be directly measured. There’s no such thing as a separate "magnetic" or "vibrational" volume that can be observed.

This is where James Clerk Maxwell—or rather, the set of thermodynamic relations that bear his name—comes into play. One of these relations states that the change in volume with temperature (thermal expansion) is equal to the change in entropy with pressure. Think of this as a change of coordinates: if we shift our perspective and control pressure instead of temperature, entropy rather than volume becomes the key variable. Entropy is a fundamental thermodynamic quantity that reflects how energy is distributed among the microscopic states of a system and, importantly, it allows us to distinguish between the vibrational and magnetic contributions to thermal expansion. Entropy increases with the level of microscopic disorder. Large atomic displacements correspond to high vibrational disorder and entropy, while the loss of spin alignment represents an increase in disorder and greater magnetic entropy.

Measuring these individual contributions is no small feat. Probing the vibrational spectrum of a material, for example, requires large particle accelerators. Controlling pressure is also significantly more challenging than simply heating a sample. It took me weeks to learn how to load samples into a diamond-anvil cell to achieve high pressures. It involved many frustrating days of staring through a microscope, trying to manipulate a sample smaller than a human hair using nothing more than a needle and a pair of tweezers. My dexterity, the fruit of years of random creative and crafting endeavors, paid off: I had an invar sample squeezed in between the tip of two opposing diamond anvils.

However, invar is also ferromagnetic. In ferromagnetic materials, the small magnetic fields created by spinning electrons are all aligned, resulting in a net macroscopic magnetization. Thermal energy excites not only atomic vibrations but also these electronic spins, which become increasingly disordered as temperature rises. This loss of magnetization has volumetric effects. To qualitatively understand this, we can rely on Pauli’s exclusion principle: Two interacting electrons cannot occupy the same energetic state. So the electronic orbitals of neighboring atoms cannot overlap if all spins are aligned. But as magnetic order relaxes with increasing temperature, this restriction also weakens, allowing atoms to move closer together and causing the material to contract.

My experimental investigation of a long-standing open question in materials science.

Electronic spins of individual atoms and the local magnetic fields they create (represented by arrows). In ferromagnetic materials, the spins are aligned at low temperatures (left), contributing to a net macroscopic magnetization. Thermal energy disrupts the spin alignment, and above the Curie temperature (right), the magnetization is lost. This magnetic disorder causes a contraction of the structure.

Stefan Lohaus, Postdoctoral Scholar at the California Institute of Technology.

After submitting an experimental proposal and waiting through the review process, I gained access to the Advanced Photon Source, a synchrotron that produces ultra-bright X-rays at Argonne National Laboratory near Chicago.

I may be biased, but our experimental setup was beautiful. Three detectors, positioned close to the sample, measured the energy exchanged between X-rays and atomic vibrations using a technique called nuclear resonant inelastic X-ray scattering. At the same time, another detector, sensitive to time-dependent changes in the X-rays interacting with magnetic spins, measured the material's local magnetization. With these two complementary techniques, we could independently track both contributions to the entropy, magnetic and vibrational, and thus, the individual pieces of the thermal expansion.

After a long and careful analysis of the data, we finally had our answer: atomic vibrations cause a substantial thermal expansion, but in invar materials, it is precisely canceled by a lattice contraction due to magnetic disordering. Beyond experimentally confirming this elegant thermodynamic explanation of the invar effect, we also discovered that vibrational and magnetic excitations are not independent. Instead, electronic spins exchange energy with atomic vibrations, which enhances the cancellation between vibrational expansion and magnetic contraction over a wider range of pressures.

This story highlights the power of studying microscopic interactions to explain macroscopic behaviors—the essence of materials science. What physical form does thermal energy take, and what atomic-scale excitations absorb that energy? The concept of entropy is the bridge between microscopic dynamics and macroscopic physical properties. And by deconstructing entropy into its vibrational and magnetic components, we were able to observe what is behind invar’s anomalous behavior. Based on what we learned from the invar effect, we could think of tailoring the amount of thermal expansion and other thermophysical properties, such as elasticity modulus, by strategically engineering their microscopic vibrational and magnetic behavior.

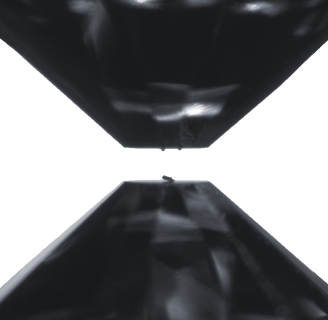
Two opposing diamonds in a diamond-anvil cell. The flat tips, called culets, are 0.3 mm across (approximately 1/100 inches). The invar sample, placed in the bottom culet, is about the width of a human hair. The two spheres on the top anvil are Ruby spheres used as reference material to monitor the pressure. Before pressuring, a metallic gasket is placed between the culets around the sample, trapping an inert gas that serves to create an isotropic (homogeneous) pressure environment at the sample.

back to research

March 4, 2025.

Experimental setup to measure the vibrational motion of atoms and the local magnetization of Invar materials at the Advanced Photon Source, a synchrotron at Argonne National Laboratory - IL. X-rays come out of the beryllium window (metallic box on the right), go through the sample inside the diamond-anvil cell (DAC), and scatter into three detectors placed closely around the DAC.

Why do some metals not expand when heated?

Overlaid snapshots of atomic positions represent the vibrational motion around their equilibrium positions in the crystal. Heat (thermal energy) induces those vibrations, which cause distortions of the structure. Larger temperatures (right figure) cause larger thermal vibrations and usually result in a volumetric expansion of the lattice.

In 1895, Swiss physicist Charles Édouard Guillaume discovered that combining two cheap and abundant metals, iron and nickel, resulted in an alloy that does not expand when heated. The volume invariance of this material with temperature earned the name invar effect. While this might not seem like the most thrilling scientific discovery, such properties are invaluable in engineered devices that require structural stability against temperature changes. Invar alloys are used in everything from large pipelines and tanks to precision instruments such as microscopes, telescopes, and orbiting satellites. By 1927, over 100 million watches had been made with Invar-like compensating springs.

Guillaume himself cleverly publicized the Invar effect to both Parisians and the academic world using none other than the newly constructed Eiffel Tower. He hung a long Invar wire from the second platform down to the ground to measure the expansion of the tower as temperatures fluctuated over several days. Since Invar remained unchanged, it served as the length reference. The technical significance of Invar, and perhaps Guillaume’s sharp publicity skills, culminated in his 1920 Nobel Prize in Physics. Because of Guillaume, Albert Einstein had to wait another year for his own Nobel, which was awarded in 1921.

Minimizing thermal expansion is technically useful, but the Invar effect also raises a puzzling scientific question: How does an alloy of two metals, both of which have substantial thermal expansion, result in a material that does not expand at all? This mystery led to thousands of scientific studies, shedding light on electronic and magnetic aspects that gave pieces of the answer. Guillaume himself suspected that magnetism played a role, as the effect vanished above the metal's Curie temperature.

As an experimental scientist, this sounds like an exciting challenge. A fundamental open question in materials science that required experimental proof was right up my alley. But I also understood why it hadn’t been done before. The volume expansion is a bulk property, meaning individual atomic-scale contributions cannot be directly measured. There’s no such thing as a separate "magnetic" or "vibrational" volume that can be observed.

This is where James Clerk Maxwell, or rather, the set of thermodynamic relations that bear his name, comes into play. One of these relations states that the change in volume with temperature (thermal expansion) is equal to the change in entropy with pressure. Think of this as a change of coordinates, if we shift our perspective and control pressure instead of temperature, entropy rather than volume becomes the key variable. Entropy is a measure of microscopic disorder, and crucially, it consists of distinct contributions from vibrations, electronic states, and magnetic spins. Large atomic displacements correspond to high vibrational disorder and entropy, while the loss of spin alignment represents an increase in disorder and larger magnetic entropy.

Measuring these individual contributions is no small feat. Probing the vibrational spectrum of a material, for example, requires large particle accelerators. Controlling pressure is also significantly more challenging than simply heating a sample. It took me weeks to learn how to load samples into a diamond-anvil cell to achieve high pressures. It involved many frustrating days of staring through a microscope, trying to manipulate a sample smaller than a human hair using nothing more than a needle and a pair of tweezers. My dexterity, the fruit of years of random creative and crafting endeavors, paid off: I had an Invar sample squeezed in between the tip of two opposing diamond anvils.

However, Invar is also a ferromagnetic. In ferromagnetic materials, the small magnetic fields created by spinning electrons are all aligned, resulting in a net macroscopic magnetization. Thermal energy excites not only atomic vibrations but also these electronic spins, which become increasingly disordered as temperature rises. This loss of magnetization has volumetric effects. A qualitative understanding comes from the Pauli exclusion principle: Two interacting electrons cannot occupy the same energetic state. So the electronic orbitals of neighboring atoms cannot overlap if all spins are aligned. But as magnetic order relaxes with increasing temperature, this restriction also weakens, allowing atoms to move closer together and causing the material to contract.

My experimental investigation of a long-standing open question in materials science.

Electronic spins of individual atoms and the local magnetic fields they create. In ferromagnetic materials, the spins are aligned at low temperatures (left), contributing to macroscopic magnetic behavior. Thermal energy disrupts the spin alignment, and above the Curie temperature (right), the net magnetization is lost. This magnetic disorder can cause a contraction of the lattice structure.

Stefan Lohaus, Postdoctoral Scholar at the California Institute of Technology.

After submitting an experimental proposal and waiting through the review process, I gained access to the Advanced Photon Source, a synchrotron that produces ultra-bright X-rays at Argonne National Laboratory near Chicago.

I may be biased, but our experimental setup was beautiful. Three detectors, positioned close to the sample, measured the energy exchanged between X-rays and atomic vibrations using a technique called nuclear resonant inelastic X-ray scattering. At the same time, another detector, sensitive to time-dependent changes in the X-rays interacting with magnetic spins, measured the material's local magnetization. With these two complementary techniques, we could independently track both contributions to the entropy, magnetic and vibrational, and thus, the individual pieces of the thermal expansion.

After a long and careful analysis of the data, we finally had our answer: atomic vibrations cause a substantial thermal expansion, but in Invar materials, it is precisely canceled by a lattice contraction due to magnetic disordering. Beyond experimentally confirming this elegant thermodynamic explanation of the Invar effect, we also discovered that vibrational and magnetic excitations are not independent. Instead, electronic spins exchange energy with atomic vibrations, enhancing the cancellation between vibrational expansion and magnetic contraction over a wider range of pressures.

This story highlights the power of studying microscopic interactions to explain macroscopic behaviors—the essence of materials science. What physical form does thermal energy take, and what atomic-scale excitations absorb that energy? The concept of entropy is the bridge between microscopic dynamics and macroscopic physical properties. And by deconstructing entropy into its vibrational and magnetic components, we were able to observe what is behind Invar’s anomalous behavior. Based on what we learned from the Invar effect, we could think of tailoring the amount of thermal expansion and other thermophysical properties, such as elasticity modulus, by strategically engineering their microscopic vibrational and magnetic behavior.

Two opposing diamonds in a diamond-anvil cell. The flat tips, called culets, are 0.3 mm across (approximately 1/100 inches). The invar sample, placed in the bottom culet, is about the width of a human hair. The two spheres on the top anvil are Ruby spheres used as reference material to monitor the pressure. Before pressuring, a metallic gasket is placed in between the culets around the sample, trapping an inert gas that serves to create an isotropic (homogeneous) pressure environment at the sample.